Article Sidebar

Download
PDF
Statistic
Read Counter : 27 Download : 2

Main Article Content

Abstract

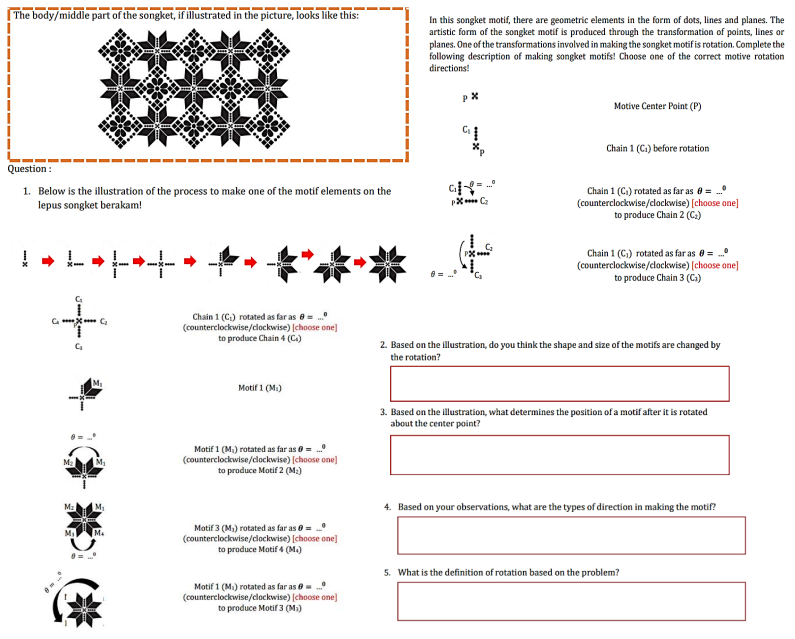
Rotation is an essential part of geometric transformations. This research aims to produce a learning trajectory of rotation material and determine the role of activities in learning the rotation which is expected to be able to support students understanding the concept of rotation learning in grade IX. The approach used is Pendidikan Matematika Realistik Indonesia (PMRI) with Lesson Study for Learning Community (LSLC) as learning system. The method used in this research is a design research type validation study. This research was conducted in limited face-to- fece meetings at secondary school number 1 Palembang during the pandemic and involved 13 students of class IX. The data were collected using video recordings and photos taken during the learning process, student interviews, student work, discussion results, and field notes at each meeting. The results of this study indicate that the learning trajectory can support the informal to formal concept of rotation, including the activity of recording the starting point and endpoint, analyzing and interpreting changes of starting and endpoints using one’s language, and writing the rotation formula. Therefore, it can be concluded that a series of activities by exploring the Palembang songket fabric motif plays a role in helping students understand the concept of rotation easily and allows them to produce rational imaginations and fun learning.


DOI: https://doi.org/10.22342/jpm.17.2.17447.189-208

Keywords

Palembang Songket Fabric Motif PMRI Rotation Design Research

Article Details

License

Copyright (c) 2023 Arika Sari, Ratu Ilma Indra Putri, Ely Susanti

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

This journal provides immediate open access to its content on the principle that making research freely available to the public supports a greater global exchange of knowledge.

All articles published Open Access will be immediately and permanently free for everyone to read and download. We are continuously working with our author communities to select the best choice of license options, Creative Commons Attribution-ShareAlike (CC BY-NC-SA).

How to Cite
Sari, A., Putri, R. I. I., & Susanti, E. (2024). Learning Rotation using the Context of Palembang Songket Fabric Motif for 3rd Grade Secondary School. Jurnal Pendidikan Matematika, 17(2), 189–208. Retrieved from https://jpm.ejournal.unsri.ac.id/index.php/jpm/article/view/171
Download Citation
Endnote/Zotero/Mendeley (RIS)
BibTeX

References

  1. Arifin. (2017). The application of the guided discovery model to the lesson study of geogebra-assisted transformation materials [in Bahasa]. APOTEMA: Jurnal Program Studi Pendidikan Matematika, 3(2), 16-25. https://doi.org/10.31597/ja.v3i2.71.
  2. Bansilal, S. & Naidoo, J. (2011). Learners engaging with transformation geometry. South African Journal of Education, 32(1, 26-39. https://doi.org/10.15700/saje.v32n1a452.
  3. D’Ambrosio, U. (1990). The history of mathematics and ethnomathematics: How a native culture intervenes in the process of learning science. Impact of Science on Society, 40(4), 369–377. https://unesdoc.unesco.org/ark:/48223/pf0000088817.
  4. Gravemeijer, K. P. E., & Cobb, P. (2006). Design research from a learning design perspective. In J. Akker, K. Gravemeijer, S. McKenney, & N. Nieveen (Eds.), Educational Design Research (pp. 45-85). Taylor and Francis Ltd. https://research.tue.nl/en/publications/design-research-from-a- learning-design-perspective.
  5. Guven, B. (2012). Using dynamic geometry software to improve eight grade students’ understanding of transformation geometry. Australasian Journal of Educational Technology, 28(2), 364-382. https://doi.org/10.14742/ajet.878.
  6. Gurbuz, K. & Durmus, S. (2009). The qualification levels of in-service elementary school teachers on the sub-learning strands, namely transformational geometry, geometric objects, pattern and tessellations. Abantİzzet Baysal University Journal of Faculty of Education, 9(1), 1-22. https://dergipark.org.tr/en/download/article-file/16660.
  7. Lestariningsih, & Mulyono, I. (2017). Learning design on transformation using batik sidoarjo motif [in Bahasa]. Journal of Education, 3(1), 83-98. https://repository.stkippgri-sidoarjo.ac.id/18/.
  8. Martin, G. (1982). Transformation Geometry: An Introduction to Symmetry. New York: Springer- Verlag. https://doi.org/10.1007/978-1-4612-5680-9.
  9. Maryati, M. & Prahmana, R. C. I. (2019). Ethnomathematics: Exploration of the Muntuk community. International Journal of Scientific & Technology Research, 8(6), 47-49. https://www.ijstr.org/final-print/june2019/Ethnomathematics-Exploration-Of-The-Muntuk-Community.pdf.
  10. Maryati, M. & Prahmana, R. C. I. (2020). Designing learning on rotation using the context of bamboo woven motif. Prima: Jurnal Pendidikan Matematika, 4(2), 100-108. https://doi.org/10.31000/prima.v4i2.2600.
  11. Mayadiana, S. D. (2009). Mathematical Critical Thinking Skills [in Bahasa]. Jakarta: Cakrawala Maha Karya.
  12. Paulsen, R. & Morris, T. (2011). Using tracing paper to teach transformation geometry". In Sherman, J. Proceedings of the Seventeenth National Congress of the Association for Mathematics Education of South Africa (AMESA): Vol. 2. Mathematics in a Globalized World (pp. 129-132). http://www.amesa.org.za/AMESA2011/Volume2.pdf.
  13. Octriana, I., Putri, R. I. I., & Nurjannah. (2019). Mathematical reasoning of students in learning uses the PMRI and LSLC approaches on number pattern material in second grade of junior high school [in Bahasa]. Jurnal Pendidikan Matematika, 13(2), 131-142. https://doi.org/10.22342/jpm.13.2.6714.131-142.
  14. Panorkou, N. & Maloney, A. (2015) Elementary students’ construction of geometric transformation reasoning in a dynamic animation environment. Constructivist Foundations 10(3), 338–347. http://constructivist.info/10/3/338.
  15. Prahmana, R. C. I. & D’ Ambrossio, U. (2020). Learning geometry and values from patterns: Ethnomathematics on the batik patterns of yogyakarta, indonesia. Journal on Mathematics Education, 11(3), 439–456. https://doi.org/10.22342/jme.11.3.12949.439-456.
  16. Putri, R. I. I. (2012). Implementation of lesson study through the PMRI approach in the statistical method course [in Bahasa]. Proceeding in The Sixteen National Conference on Mathematics 2012, pp. 450-460. Jatinangor: Universitas Padjajaran.
  17. Putri, R. I. I., & Zulkardi. (2017). Fraction in shot-put: A learning trajectory. AIP Conference Proceedings, 1868, 050005. https://doi.org/10.1063/1.4995132.
  18. Putri, R. I. I., & Zulkardi. (2019). Designing jumping task on percent using PMRI and collaborative learning. International Journal on Emerging Mathematics Education, 3(1), 1-8. http://dx.doi.org/10.12928/ijeme.v3i1.12208.
  19. Rahmawati, K. (2016). Development of MOODLE-Based e-learning as a social studies learning resource for class vii junior high school sub-theme of economic activities and utilization of natural resources [in Bahasa]. Undergraduate Thesis. Yogyakarta: Yogyakarta State University. https://eprints.uny.ac.id/40107/.
  20. Risdiyanti, I. & Prahmana, R. C. I. (2018). Ethnomathematics: Exploration in Javanese culture. Journal of Physics: Conference Series, 943(1), 012032. https://doi.org/10.1088/1742- 6596/943/1/012032.
  21. Risdiyanti, I. & Prahmana, R. C. I. (2020). The learning trajectory of number pattern learning using barathayudha war stories and uno stacko. Journal on Mathematics Education, 11(1), 157-166. https://doi.org/10.22342/jme.11.1.10225.157-166.
  22. Sari, A., & Putri, R. I. I. (2021). inductive reasoning ability of students using the Palembang songket fabric context in rotational learning in grade IX. Jurnal Pendidikan Matematika, 16(1), 57-72. https://doi.org/10.22342/jpm.16.1.14304.57-72.
  23. Sato, M. (2014). Reforming Schools of Community Learning Concepts and Practices [in Bahasa]. Jakarta: Pelita.
  24. Sembiring, R. K., Hadi, S. & Dolk, M. (2008). Reforming mathematics learning in Indonesian classrooms through RME. ZDM Mathematics Education, 40, 927–939. https://doi.org/10.1007/s11858-008-0125-9.
  25. Thaqi, X. & Gimenez, J. (2011). Prospective teacher's understanding of geometric transformation. 12th International Congress on Mathematical Education. Seoul: TSG10. bit.ly/467yNSs.
  26. Utami, I. W. P., & Nafi’ah, M. U. (2016). A model of microteaching lesson study implementation in the prospective history teacher education. Journal of Education and Practice, 7(27), 10-14. https://files.eric.ed.gov/fulltext/EJ1115840.pdf.
  27. Wijaya, A. (2012). Realistic Mathematics Education: Alternative Approaches to Learning Mathematics [in Bahasa]. Yogyakarta: Graha Ilmu. https://www.researchgate.net/publication/316598093_Pendidikan_Matematika_Realistik_Suatu_Alternatif_Pendekatan_Pembelajaran_Matematika.
  28. Zembat, I. (2013). Geometrik dönüşümlerden dönme ve özellikleri. Ankara: Pegem Akademi Yayıncılık.https://www.researchgate.net/publication/353954140_Geometrik_donusumlerden_donme_ve_ozellikleri.
  29. Zulkardi & Putri, R. I. I. (2019). New School Mathematics Curricula, PISA and PMRI in Indonesia. In: Vistro-Yu, C., Toh, T. (eds) School Mathematics Curricula. Mathematics Education – An Asian Perspective. Springer, Singapore. https://doi.org/10.1007/978-981-13-6312-2_3.
  30. Zulkardi. (2002). Developing a learning environment on realistic mathematics education for indonesian student teachers. Doctoral Thesis. Enschede: Twente University. https://research.utwente.nl/en/publications/developing-a-learning-environment-on-realistic- mathematics-educat.

Most read articles by the same author(s)

1 2 3 > >> 

Similar Articles

<< < 1 2 3 4 5 6 7 8 9 10 > >> 

You may also start an advanced similarity search for this article.