Article Sidebar

Download
PDF
Statistic
Read Counter : 23 Download : 1

Main Article Content

Abstract

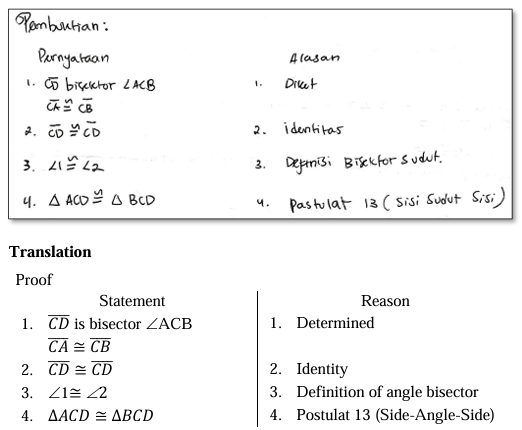
Reasoning  that  is  constructed  from  remembering  is  imitative  reasoning,  while  the  opposite  is  creative reasoning. This study aims to explore creative mathematical reasoning in solving geometric problems. Mathematical creative reasoning is reasoning that contains elements of novelty, plausibility, and mathematical foundation. This type of research is descriptive qualitative, which is explorative. The research subjects were the first-semester student in the mathematics education study program with 32 students. The results showed that from 32 students, there was only one student identified as having creative mathematical reasoning in solving geometry problems. Creative mathematical reasoning can be identified when the subject is able to reason algorithmically but is aware of problems so they cannot be resolved algorithmically so that they must form new reasoning, which consists of novelty, plausibility, and mathematical foundation. Creative mathematical reasoning arises after students make an algorithmic reasoning process, but find no solution. Novelty is the weakest indicator of creative mathematical reasoning, so it requires scaffolding to bring it up.

Keywords

Creative Mathematical Reasoning Problem Solving Geometry

Article Details

License

Copyright (c) 2020 PDF

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

This journal provides immediate open access to its content on the principle that making research freely available to the public supports a greater global exchange of knowledge.

All articles published Open Access will be immediately and permanently free for everyone to read and download. We are continuously working with our author communities to select the best choice of license options, Creative Commons Attribution-ShareAlike (CC BY-NC-SA).

How to Cite
Masfingatin, T., Murtafiah, W., & Maharani, S. (2020). Exploration of Creative Mathematical Reasoning in Solving Geometric Problems. Jurnal Pendidikan Matematika, 14(2), 155–168. Retrieved from https://jpm.ejournal.unsri.ac.id/index.php/jpm/article/view/138
Download Citation
Endnote/Zotero/Mendeley (RIS)
BibTeX

References

  1. Adawiyah, R., Muin, A., & Khairunnisa, K. (2017). Mathematical Inductive-creative reasoning, a theoretical study. Advances in Social Science, Education and Humanities Research (ASSEHR), 57, 247–251. https:// dx.doi.org/10.2991/icmsed-16.2017.53.
  2. Bergqvist, T., & Lithner, J. (2012). Mathematical reasoning in teachers’ presentations. Journal of Mathematical Behavior, 31(2), 252–269. https://doi.org/10.1016/j.jmathb.2011.12.002.
  3. Birkeland, A. (2019). Pre-service teachers’ mathematical reasoning - how can it be developed?. The Mathematics Enthusiast, 16(1–3), 579–596. Retrieved from https://scholarworks.umt.edu/tme/vol16/iss1/29.
  4. Boesen, J., Lithner, J., & Palm, T. (2010). The relation between types of assessment tasks and the mathematical reasoning students use. Educ Stud Math, 75, 89–105. https://doi.org/10.1007/s10649-010-9242-9.
  5. Darta, & Saputra, J. (2018). Indicators that influence prospective mathematics teachers representational and reasoning abilities. Journal of Physics: Conference Series, 948(1). https://doi.org/10.1088/1742-6596/948/1/012053.
  6. Handayani, A. D. (2013). Creative mathematical reasoning [in Bahasa]. Jurnal Pengajaran MIPA, 18(2), 161–166. https://doi.org/http://dx.doi.org/10.18269/jpmipa.v18i2.
  7. Hendriana, H., Prahmana, R. C. I., & Hidayat, W. (2018). Students’ performance skills in creative mathematical reasoning. Infinity Journal, 7(2), 83. https://doi.org/10.22460/infinity.v7i2.p83-96.
  8. Hidayat, W., Wahyudin, & Prabawanto, S. (2018). Improving students’ creative mathematical reasoning ability students through adversity quotient and argument driven inquiry learning. Journal of Physics: Conference Series, 948(1). https://doi.org/10.1088/1742-6596/948/1/012005.
  9. Hidayat, W. (2017). Adversity quotient and creative mathematical reasoning of senior high school students in argument-driven inquiry learning on derived functions material [in Bahasa]. KALAMATIKA Jurnal Pendidikan Matematika, 2(1), 15. https://doi.org/10.22236/kalamatika.vol2no1.2017pp15-28.
  10. Hidayat, W., Herdiman, I., Aripin, U., Yuliani, A., & Maya, R. (2018). Adversity Quotient (AQ) and creative mathematical reasoning of pre-service teacher [in Bahasa]. Jurnal Elemen, 4(2), 230. https://doi.org/10.29408/jel.v4i2.701.
  11. Jäder, J. (2019). Task design with a focus on conceptual and creative challenges. In Eleventh congress of the european society for research in mathematics education, Utrecht University Netherlands: Utrecht (p. hal-02423408).
  12. Jane, M . (2006). Developing geometric reasoning. Washington DC: GED Mathematics Training Institute.
  13. Jonsson, B., Kulaksiz, Y. C., & Lithner, J. (2016). Creative and algorithmic mathematical reasoning: effects of transfer-appropriate processing and effortful struggle. International Journal of Mathematical Education in Science and Technology, 47(8), 1206–1225. https://doi.org/10.1080/0020739X.2016.1192232.
  14. Jonsson, B., Norqvist, M., Liljekvist, Y., & Lithner, J. (2014). Learning mathematics through algorithmic and creative reasoning. Journal of Mathematical Behavior, 36, 20–32. https://doi.org/10.1016/j.jmathb.2014.08.003.
  15. Lawson, M. J., & Chinnappan, M. (2000). Knowledge connectedness in geometry problem solving. Journal for Research in Mathematics Education, 31(1), 26–43. https://doi.org/10.2307/749818.
  16. Lithner, J. (2008). A Research Framework for Creative and Imitative Reasoning. Educational Studies in Mathematics, 67(3), 255–276. https://doi.org/10.1007/sl0649-007-9104-2.
  17. Lithner, J. (2017). Principles for designing mathematical tasks that enhance imitative and creative reasoning. ZDM - Mathematics Education, 49(6), 937–949. https://doi.org/10.1007/s11858-017-0867-3.
  18. Masfingatin, T., Murtafiah, W., & Krisdiana, I. (2018). Mathematics pre-service teacher ability in solving problems of geometry theorema proof [in Bahasa]. Jurnal Mercumatika: Jurnal Penelitian Matematika dan Pendidikan Matematika, 2(2), 41–50. https://dx.doi.org/10.26486/jm.v2i2.272.
  19. Mullis, I. V. S., Martin, M. O., & Foy, P. (2015). TIMSS 2015 international results in mathematics. Boston: International Association for the Evaluation of Educational Achievement.
  20. NCTM. (2000). Six principles for school mathematics. In National Council of Teachers of Mathematics (pp. 1–6).
  21. Norqvist, M., Jonsson, B., Lithner, J., Qwillbard, T., & Holm, L. (2019). Investigating algorithmic and creative reasoning strategies by eye tracking. Journal of Mathematical Behavior, 55(April), 100701. https://doi.org/10.1016/j.jmathb.2019.03.008.
  22. Nursyahidah, F., Saputro, B. A., & Prayitno, M. (2016). Mathematical reasoning ability of junior high school students in learning of lines and angles with geogebra [in Bahasa]. Suska Journal of Mathematics Education, 2(1), 13-19.
  23. Octriana, I., Putri, R. I. I., & Nurjannah, N. (2019). Students' mathematical reasoning in learning of number patterns using PMRI and LSLC [in Bahasa]. Jurnal Pendidikan Matematika, 13(2),131–142. https://doi.org/10.22342/jpm.13.2.6714.131-142.
  24. Olsson, J. (2017). GeoGebra, enhancing creative mathematical reasoning. Institutionen för tillämpad utbildningsvetenskap. Retrieved from http://www.diva- portal.org/smash/get/diva2:1085687/FULLTEXT01.pdf.
  25. Permendikbud. (2016). Minister of Education and Culture Regulation Number 21 of 2016 concerning Basic and Secondary Education Content Standards [in Bahasa]. Jakarta: Permendikbud.
  26. Reiss, K. M., Heinze, A., Renkl, A., & Groß, C. (2008). Reasoning and proof in geometry: Effects of a learning environment based on heuristic worked-out examples. ZDM - International Journal on Mathematics Education, 40(3), 455–467. https://doi.org/10.1007/s11858-008-0105-0.
  27. Rohana, R., & Ningsih, Y. L. (2019). Students’ statistical reasoning in statistics method course. Jurnal Pendidikan Matematika, 14(1), 81–90. https://doi.org/10.22342/jpm.14.1.6732.81-90. Schoenfeld, A. H. (1985). Mathematical problem solving. Orlando: Fla: Academic Press.
  28. Sentosa, C. A. H. (20013). Overcoming student difficulties when conducting formal mathematical proof [in Bahasa]. Jurnal Pengajaran MIPA, 18(2), 152–160. https://doi.org/http://dx.doi.org/10.18269/jpmipa.v18i2.
  29. Siregar, N. (2018). Reviewing mathematical reasoning abilities of junior high school students through interviews based on geometry task [in Bahasa]. Mosharafa: Jurnal Pendidikan Matematika, 5(2), 128–137. https://doi.org/10.31980/mosharafa.v5i2.268.
  30. Sukirwan, Darhim, D., & Herman, T. (2018). Analysis of students’ mathematical reasoning. Journal of Physics: Conference Series, 948(1). https://doi.org/10.1088/1742-6596/948/1/012036.
  31. Verner, I., Massarwe, K., & Bshouty, D. (2019). Development of competencies for teaching geometry through an ethnomathematical approach. Journal of Mathematical Behavior, (May), 100708. https://doi.org/10.1016/j.jmathb.2019.05.002.