Article Sidebar

Download
Statistic
Read Counter : 0

Main Article Content

Abstract

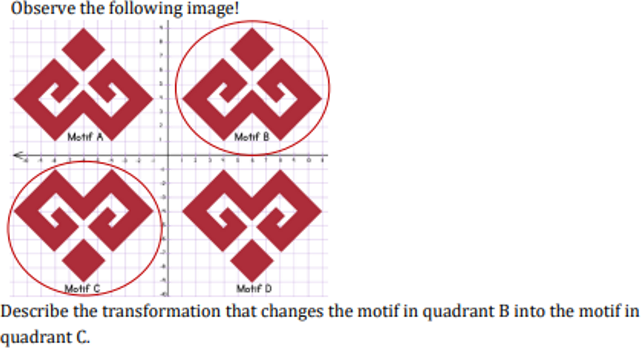
This study addresses the limited use of the South Sumatera Songket motifs for supporting students’ proving process in the learning of reflection material. It aims to determine the role of South Sumatera Songket Motifs in students’ proving process in reflection learning. The study employed a validation approach within the Design Research framework, involving 30 students from one public junior high school in Palembang. The validation study comprised three stages: preparing for the experiment, the design experiment (including a preliminary teaching experiment and a teaching experiment), and retrospective analysis. Activities in the experiment included: 1) introducing the Songket motifs and having students identify lines of symmetry, 2) guiding students to reflect on the motifs using various axes, 3) engaging students in proving the congruence properties of the reflected motif, and 4) conducting group discussions to refine their reasoning and articulation of geometric concepts. The activities were designed using indicators adapted from Habermas’ Construct of Rationality to support the proving process in reflection material. The results from the learning experiment indicate that the provided questions support students’ proving process, guiding them from visual recognition to mathematical reasoning through structured activities.

Keywords

Cultural Relevance Design Research Proving Process Reflection South Sumatera Songket Motif

Article Details

License

Copyright (c) 2025 Arika Sari, Ratu Ilma Indra Putri, Zulkardi, Rully Charitas Indra Prahmana

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

This journal provides immediate open access to its content on the principle that making research freely available to the public supports a greater global exchange of knowledge.

All articles published Open Access will be immediately and permanently free for everyone to read and download. We are continuously working with our author communities to select the best choice of license options, Creative Commons Attribution-ShareAlike (CC BY-NC-SA).

How to Cite
Sari, A., Putri, R. I. I., Zulkardi, & Prahmana, R. C. I. (2025). The South Sumatera Songket Motifs for Supporting Students’ Proving Process in Learning Reflection. Mathematics Education Journal, 19(2), 343–364. https://doi.org/10.22342/mej.v19i2.pp343-364
Download Citation
Endnote/Zotero/Mendeley (RIS)
BibTeX

References

  1. Akarsu, M., & İler, K. (2024). A research on transformation geometry: Answered and unanswered questions. İnönü Üniversitesi Eğitim Fakültesi Dergisi, 25(3), 1240-1264. https://doi.org/10.17679/inuefd.1453349
  2. Andriyani, R., Rahman, R., Irawati, R., Mutaqin, E. J., & Kamis, N. (2023). Exploration of Batik Jambi on learning transformation geometry. Prima: Jurnal Pendidikan Matematika, 7(1), 89–99. https://doi.org/10.31000/prima.v7i1.7305
  3. Aktaş, G., & Ünlü, M. (2017). Understanding of eighth-grade students about transformation geometry: Perspectives on students’ mistakes. Journal of Education and Training Studies, 5(5), 103–119. https://doi.org/10.11114/JETS.V5I5.2254
  4. Aras, A., Prahmana, R. C. I., Buhaerah, B., Busrah, Z., Jumaisa, J., & Setialaksana, W. (2023). Reflection learning innovation in the context of the lipa sabbe bunga caramming motif. Al-Jabar: Jurnal Pendidikan Matematika, 14(1). https://doi.org/10.24042/ajpm.v14i1.15912
  5. Bakker, A. (2018). Design research in education: A practical guide for early career researchers. Routledge. https://doi.org/10.4324/9780203701010
  6. Baroa, J. (2025). Students’ common errors in geometric proving: Basis for an enhancement program. International Journal for Multidisciplinary Research, 7(2). https://doi.org/10.36948/ijfmr.2025.v07i02.37424
  7. Büscher, C. (2024). Adapting Habermas’ construct of communicative rationality into a framework for analyzing students’ statistical literacy. Educational Studies in Mathematics, 117, 121-141. https://doi.org/10.1007/s10649-024-10325-5
  8. Götz, D., & Gasteiger, H. (2018). Typical student errors in axis mirroring - An analysis of student responses [in German]. In Fachgruppe Didaktik der Mathematik der Universität Paderborn (Eds.), Beiträge zum Mathematikunterricht 2018 (pp. 631–634). WTM.
  9. Götz, D., & Gasteiger, H. (2022). Reflecting geometrical shapes: Approaches of primary students to reflection tasks and relations to typical error patterns. Educational Studies in Mathematics, 111(1), 47–71. https://doi.org/10.1007/s10649-022-10145-5
  10. Guven, B. (2012). Using dynamic geometry software to improve eight grade students' understanding of transformation geometry. Australasian Journal of Educational Technology, 28(2), 364-382. https://doi.org/10.14742/ajet.878
  11. Haj-Yahya, A. (2021a). Can a number of diagrams linked to a proof task in 3D geometry improve proving ability? Mathematics Education Research Journal, 35, 215-236. https://doi.org/10.1007/s13394-021-00385-8
  12. Haj-Yahya, A. (2021b). Students' conceptions of the definitions of congruent and similar triangles. International Journal of Mathematical Education in Science and Technology, 53(10), 2703-2727. https://doi.org/10.1080/0020739X.2021.1902008
  13. Hollebrands, K. F. (2003). High school student’s understanding of geometric transformation in the context of a technological environment. The Journal of Mathematical Behavior, 22(1), 1-19. https://doi.org/10.1016/S0732-3123(03)00004-X
  14. Indahwati, R. (2023). Students’ difficulties in transformation geometry courses viewed from visualizer-verbalizer cognitive style. Sigma, 9(1). https://doi.org/10.53712/sigma.v9i1.1993
  15. Kim, Y., & Shin, B. (2023). An analysis of students’ communication in lessons for the geometric similarity using AlgeoMath. Korean School Mathematics Society, 26(2), Article 003. https://doi.org/10.30807/ksms.2023.26.2.003
  16. Knuchel, C. (2004). Teaching symmetry in the elementary curriculum. The Montana Mathematics Enthusiast, 1(1), 3–8. https://doi.org/10.54870/1551-3440.1001
  17. Leonard, J., & Guha, S. (2002). Creating cultural relevance in teaching and learning mathematics. Teaching Children Mathematics, 9(2), 114-118. https://doi.org/10.5951/TCM.9.2.0114
  18. Nurazizah, I., & Zulkardi, Z. (2022). Students’ mathematical reasoning ability in solving PISA-like mathematics problem COVID-19 context. Jurnal Elemen, 8(1), 250-262. https://doi.org/10.29408/jel.v8i1.4599
  19. Nurhikmayati, I., Jatisunda, M. G., & Ratnawulan, N. (2022). The practice of reflection based on didactical design research: An analysis of the geometry transformation material. JTAM (Jurnal Teori dan Aplikasi Matematika), 6(3), 265–277. https://doi.org/10.31764/jtam.v6i3.8441
  20. Noto, M., Priatna, N., & Dahlan, J. A. (2019). Mathematical proof: The learning obstacles of pre-service mathematics teachers on transformation geometry. Journal on Mathematics Education, 10(1), 117–126. https://doi.org/10.22342/JME.10.1.5379.117-126
  21. P, S., & P, S. S. K. (2022). A systematic investigation on geometric transformation. 2022 Third International Conference on Intelligent Computing Instrumentation and Control Technologies (ICICICT), 343–350. https://doi.org/10.1109/ICICICT54557.2022.9917968
  22. Pauji, I., Hadi, H., & Juandi, D. (2023). Systematic literature review: Analysis of learning obstacles in didactical design research on geometry material. Jurnal Cendekia: Jurnal Pendidikan Matematika, 7(3). https://doi.org/10.31004/cendekia.v7i3.2474
  23. Putri, R. I. I., Setyorini, N. P., Meitrilova, A., Permatasari, R., Saskiyah, S. A., & Nusantara, D. S. (2021). Designing a healthy menu project for indonesian junior high school students. Journal on Mathematics Education, 12(1), 133-146. https://doi.org/10.22342/jme.11.1.9786.135-144
  24. Sari, A., Putri, R. I. I., Zulkardi, Z., & Prahmana, R. C. I. (2024). Ethnomathematics in Indonesian woven fabric: The promising context in learning geometry. Mathematics Teaching Research Journal, 16(5), 157-185. https://files.commons.gc.cuny.edu/wp-content/blogs.dir/34462/files/2024/11/7-Sari-et-al-Ethnomathematics-in-Indonesian-Woven-Fabric.pdf
  25. Shen, P., Li, R., Wang, B., & Liu, L. (2023). Scratch-based reflection art via differentiable rendering. ACM Transactions on Graphics, 42(4), 1–12. https://doi.org/10.1145/3592142
  26. Uygun, T. & Akyuz, D. (2019). Developing subject matter knowledge through argumentation. Internationa lJournal of Research in Education and Science (IJRES), 5(2), 532–547. https://ijres.net/index.php/ijres/article/view/568
  27. Velichová, D. (2021). The mysterious synergy of geometry and art. In L. Y. Cheng (Ed.), ICGG 2020 – Proceedings of the 19th International Conference on Geometry and Graphics (Advances in Intelligent Systems and Computing, Vol. 1296). Springer. https://doi.org/10.1007/978-3-030-63403-2_24
  28. Williford, H. J (1972). A study of transformational geometry instruction in the primary grades. Journal for Research in Mathematics Education, 3(4), 260-271. https://doi.org/10.2307/748493
  29. Yurmalia, D., & Herman, T. (2021). Student visualization in solving geometry problems: The case of reflection [Paper presentation]. In Journal of Physics: Conference Series, 1806(1). International Conference on Mathematics and Science Education (ICMScE), Jawa Barat, Indonesia. https://doi.org/10.1088/1742-6596/1806/1/012052
  30. Zhuang, Y., & Conner, A. (2022). Teachers’ use of rational questioning strategies to promote student participation in collective argumentation. Educational Studies in Mathematics, 111(3), 345–365. https://doi.org/10.1007/s10649-022-10160-6

Most read articles by the same author(s)

1 2 3 4 5 6 7 > >> 

Similar Articles

1 2 3 4 5 6 7 8 9 10 > >> 

You may also start an advanced similarity search for this article.