Article Sidebar

Article 01
Download
PDF
Statistic
Read Counter : 6 Download : 1

Main Article Content

Abstract

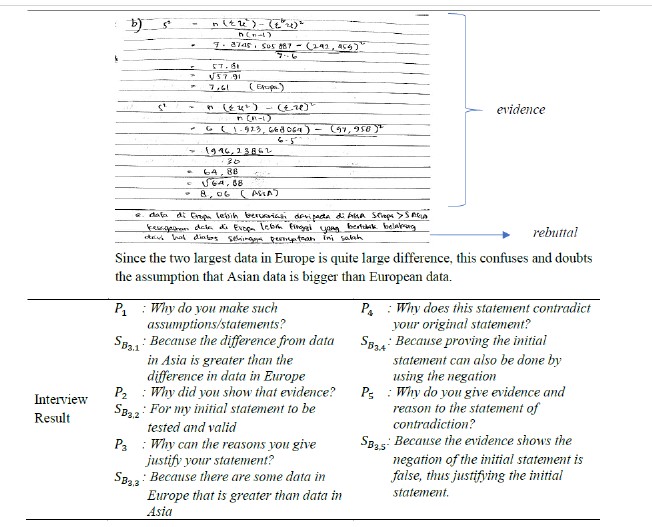
Evaluation of the argumentation structure is needed to check the quality of student argumentation to produce appropriate problem-solving. Such evaluation can be carried out by identifying the constituent components of the argument. This study aims to describe the structure of student argumentation in solving statistical problems based on the Adversity Quotient (AQ). This qualitative descriptive type of research involved 52 students who were taking statistical methods courses. Participants were classified into three Categories of Adversity Quotient based on the results of the ARP (Adversity Response Profile) questionnaire. Data were obtained using statistical problem tests and interviews. The results showed that students with the AQ Climber category were able to meet all the constituent components of argumentation when solving statistical problems. AQ Camper-type students are only able to meet three components, namely claims, evidence, and reasoning. Meanwhile, students with the AQ Quitter type are only able to fulfill one component, namely claims. Based on the results of the study, the level of Adversity Quotient determines the quality of the student's argumentation structure when solving statistical problems.

DOI: https://doi.org/10.22342/jpm.16.2.16633.121-140

Keywords

Argumentation Statistics Adversity Quotient

Article Details

License

Copyright (c) 2022 Iffanna Fitrotul Aaidati, Subanji, I Made Sulandra, Hendro Permadi

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

This journal provides immediate open access to its content on the principle that making research freely available to the public supports a greater global exchange of knowledge.

All articles published Open Access will be immediately and permanently free for everyone to read and download. We are continuously working with our author communities to select the best choice of license options, Creative Commons Attribution-ShareAlike (CC BY-NC-SA).

How to Cite
Aaidati, I. F., Subanji, Sulandra, I. M., & Permadi, H. (2024). Student Argumentation Structure in Solving Statistical Problems Based on Adversity Quotient. Jurnal Pendidikan Matematika, 16(2), 121–140. Retrieved from https://jpm.ejournal.unsri.ac.id/index.php/jpm/article/view/185
Download Citation
Endnote/Zotero/Mendeley (RIS)
BibTeX

References

  1. Berland, L. K., & McNeill, K. L. (2010). A learning progression for scientific argumentation: Understanding student work and designing supportive instructional contexts. Science Education, 94(5), 765–793. https://doi.org/10.1002/sce.20402
  2. Bluman, G. A. (2009). Elementary Statistics: a step by step approach 7th Edition. New York: McGraw- Hill.
  3. Chasanah, A. N., Wicaksono, A. B., Nurtsaniyah, S., & Utami, R. N. (2020). Analyze The Students’s Mathematics Literacy Abilities in Inferenstial Statistics Subject Based on the Learning Styles. Edumatica Jurnal Pendidikan Matematika, 10(2). https://doi.org/https://doi.org/10.22437/edumatica.v10i2.10621
  4. Cross, D., Taasoobshirazi, G., Hendricks, S., & Hickey, D. T. (2008). Argumentation: A strategy for improving achievement and revealing scientific identities. International Journal of Science Education, 30(6), 837–861. https://doi.org/10.1080/09500690701411567
  5. Faizah, L., Probosari, R. M., & Karyanto, P. (2018). Application of Problem Based Learning to Improve Oral Argumentation Skills of Class XI Students on Biological Learning. Jurnal Biotek, 6(2). https://doi.org/https://doi.org/10.24252/jb.v6i2.6395
  6. Fischer, F., Kollar, I., Ufer, S., Sodian, B., & Hussmann, H. (2014). Scientific Reasoning and Argumentation: Advancing an Interdiciplinary Research Agenda in Education. Frontline Learning Research, 5, 28–45. https://doi.org/https://doi.org/10.14786/flr.v2i2.96
  7. Hadi, S., Gunawan, I., & Dalle, J. (2018). Statistical Inferential Theory and Its Application.
  8. Haerudin, H., & Nur, I. R. D. (2020). Analysis of Student Difficulties in Inferential Statistics Courses. Lebesgue: Jurnal Ilmiah Pendidikan Matematika, Matematika, Dan Statistika, 1(3). https://doi.org/10.46306/lb.v1i3
  9. Hakim, F., & Murtafiah, M. (2020). Adversity Quotient and Resilience in Mathematical Proof Problem- Solving Ability. MaPan: Jurnal Matematika Dan Pembelajaran, 8(1), 87. https://doi.org/10.24252/mapan.2020v8n1a7
  10. Heng, L. L., Surif, J., & Seng, C. H. (2014). Individual versus group argumentation: Student’s performance in a Malaysian context. International Education Studies, 7(7), 109–124. https://doi.org/10.5539/ies.v7n7p109
  11. Herhyanto, N. (2016). Education Statistics. Tangerang Selatan: Universitas Terbuka.
  12. Hidayat, W. (2017). Adversity Quotient and Mathematical Creative Reasoning of High School Students In Argument Driven Learning. Kalamatika:Jurnal Pendidikan Matematika, 2(1), 15–28. https://doi.org/https://doi.org/10.22236/KALAMATIKA.vol2no1.2017pp15-28
  13. Hidayat, W., & Sariningsih, R. (2018). Mathematical Problem-Solving Ability and Adversity Quotient of Junior High School Students Through Open Ended Learning. JNPM (Jurnal Nasional Pendidikan Matematika), 2(1), 109–118.
  14. Hidayat, W., Wahyudin, & Prabawanto, S. (2018a). The mathematical argumentation ability and adversity quotient (AQ) of pre-service mathematics teacher. Journal on Mathematics Education, 9(2), 239–248. https://doi.org/10.22342/jme.9.2.5385.239-248
  15. Hidayat, W., Wahyudin, W., & Prabawanto, S. (2018b). Improving students’ creative mathematical reasoning ability students through adversity quotient and argument driven inquiry learning. Journal of Physics: Conference Series, 948(1). https://doi.org/10.1088/1742-6596/948/1/012005
  16. Hidayat, Wahyu., Herdiman, Indri., Aripin, Usman., Yuliani, Anik., & Maya, R. (2018). Adversity Quotient (AQ) and Mathematical Creative Reasoning of Prospective Teacher Students. Jurnal Elemen, 4(2), 230. https://doi.org/10.29408/jel.v4i2.701
  17. Ho, H. Y., Chang, T. L., Lee, T. N., Chou, C. C., Hsiao, S. H., Chen, Y. H., & Lu, Y. L. (2019). Above-
  18. and below-average Students Think Differently: Their scientific argumentation patterns. Thinking Skills and Creativity. https://doi.org/10.1016/j.tsc.2019.100607
  19. Inglis, M., Mejia-Ramos, J. P., & Simpson, A. (2007). Modelling mathematical argumentation: The importance of qualification. Educational Studies in Mathematics, 66(1), 3–21. https://doi.org/10.1007/s10649-006-9059-8
  20. Khumairoh, B., Amin, S. M., & Wijayanti, P. (2020). Proportional Reasoning of Middle School Students in Solving Mathematical Problems Viewed from Adversity Quotient. PEDAGOGIA: Jurnal Pendidikan, 9(1). https://doi.org/10.21070/pedagogia.v%vi%i.259
  21. Krawczyk, D. C. (2018). Introduction to Reasoning. In Reasoning (pp. 1–11). Elsevier. https://doi.org/10.1016/b978-0-12-809285-9.00001-6
  22. Lizotte, D. J., Harris, C. J., Mcneill, K. L., Marx, R. W., & Krajcik, J. (2003). Usable Assessments Aligned with Curriculum Materials: Measuring Explanation as a Scientific Way of Knowing. In Annual meeting of the American Educational Research Association.
  23. Lusiana, R., Suprapto, E., Sukristini, I., Studi, P., & Matematika, P. (2021). The Effectiveness of Problem Based Learning (PBL) on Mathematics Learning Achievement in terms of Student Adversit. Edumatica Jurnal Pendidikan Matematika, 11(2). https://doi.org/https://doi.org/10.22437/edumatica.v11i02.7670
  24. McNeill, K. L., & Krajcik, J. (2011). Supporting grade 5-8 students in constructing explanation in science. London, UK: Pearson.
  25. Mcneill, B. K. L., & Martin, D. M. (2011). Demystifying data during a unit on simple machines.
  26. National Research Council (NRC 1996).
  27. McNeill, K., & Krajcik, J. (2008). Inquiry and scientific explanations: Helping students use evidence and reasoning. In Science as Inquiry in The Secondary Setting.
  28. McNeill, K. L., & Krajcik, J. (2009). Synergy between teacher practices and curricular scaffolds to support students in using domain-specific and domain-general knowledge in writing arguments to explain phenomena. Journal of the Learning Sciences, 18(3), 416–460. https://doi.org/10.1080/10508400903013488
  29. McNeill, K. L., & Martin, D. M. (2011). Claims, Evidence, and Reasoning. Science and Childern. www.nsta.org/SC1104
  30. Mercier, H., & Sperber, D. (2011). Why do humans’ reason? Arguments for an argumentative theory.
  31. Behavioral and Brain Sciences, 34(2), 57–74. https://doi.org/10.1017/S0140525X10000968ï
  32. Mercier, H., & Sperber, D. (2013). Why do humans’ reason? Arguments for an argumentative theory. Behavioral and Brain Sciences, Cambridge University Press (CUP), 34(2), 57–74. https://doi.org/10.1017/S0140525X10000968
  33. Mueller, M., Yankelewitz, D., & Maher, C. (2012). A framework for analyzing the collaborative construction of arguments and its interplay with agency. Educational Studies in Mathematics, 80(3), 369–387. https://doi.org/10.1007/s10649-011-9354-x
  34. Muratsu, K., Inagaki, S., Yamaguchi, E., Yamamoto, T., Sakamoto, M., & Kamiyama, S. (2015). An Evaluation of Japanese Elementary Students’ Understanding of the Criteria for Rebuttals in Argumentation. Procedia - Social and Behavioral Sciences, 167, 91–95. https://doi.org/10.1016/j.sbspro.2014.12.648
  35. Nordin, A. K., & Björklund, B. L. (2018). A framework for identifying mathematical arguments as supported claims created in day-to-day classroom interactions. Journal of Mathematical Behavior, 51, 15–27. https://doi.org/10.1016/j.jmathb.2018.06.005
  36. Putri, M. E. (2017). Student Creative Thinking Process in Solving Open-Ended Problems Reviewed from Adversity Quotient (AQ) Students. State University of Malang: Unpublished Thesis.
  37. Rohana, R., & Yunika, L. N. (2020). Students’ Statistical Reasoning in Statistics Method Course, Online Submission. Jurnal Pendidikan Matematika, 14(1), 81–90. https://doi.org/https://doi.org/10.22342/jpm.14.1.6732.81-90
  38. Sadieda, L. U. (2019). Ability to argue students through inductive thinking models with probing- prompting learning methods. Pythagoras: Jurnal Pendidikan Matematika, 14(1), 23–32. https://doi.org/10.21831/pg.v14i1.24038
  39. Sanit, I. N., & Sulandra, I. M. (2019). Student Algebraic Reasoning Profiles in Solving Math Problems Reviewed from Adversity Quotient. Jurnal Pendidikan: Teori, Penelitian, Dan Pengembangan, 4(9), 1213–1221. http://dx.doi.org/10.17977/jptpp.v4i9.12711
  40. Santoso, K. F., Budiarto, M. T., & Sulaiman, R. (2019). Argumentation in Covariational Reasoning: Middle School Student’s Solving Covariation Problem with Different Cognitive Style. International Conference on Science, Technology, Education, Arts, Culture, and Humanity (STEACH), 277, 27–31. https://doi.org/10.2991/steach-18.2019.6
  41. Septiana, A. (2019). Mathematics Adversity Quotient Degree in Students of IAIN Curup Mathematics Tadris Study Program. Academic Journal of Math, 01(01), 51–62. https://doi.org/http://dx.doi.org/10.29240/ja.v1i1.826
  42. Stoltz, P. G. (1997). Adversity Quotient: Turning Obstacles into Opportunities. John Wiley & Sons. Subanji. (2011). The theory of pseudo-thinking of kovariasional reasoning. Malang: UM Press.
  43. Sugiyono. (2013). Qualitative Quantitative Research Methods and R&D. Bandung: Alfabeta.
  44. Supardi U.S., S. U. S. (2015). The Influence of Adversity Qoutient on Math Learning Achievement. Formatif: Jurnal Ilmiah Pendidikan MIPA, 3(1), 61–71. https://doi.org/10.30998/formatif.v3i1.112
  45. Sutini, S., Aaidati, I. F., & Kusaeri, K. (2020). Identifying the structure of students’ argumentation in covariational reasoning of constructing graphs. Beta: Jurnal Tadris Matematika, 13(1), 61–80. https://doi.org/10.20414/betajtm.v13i1.374
  46. Umah, U., Asari, A. R., & Sulandra, I. M. (2016). Structure Of Argumentation Reasoning Kovariasional Students Class Viiib Mtsn 1 Kediri. JMPM: Jurnal Matematika dan Pendidikan Matematika, 1(1), 1. https://doi.org/10.26594/jmpm.v1i1.498
  47. Yani, M., Ikhsan, M., & Marwan. (2016). First High School Students' Thought Process In Solving Math Problems Based on Polya Steps Reviewed from Adversity Quotient. Jurnal Pendidikan Matematika, 10(1), 43–58.